The Fibonacci Sequence’s Endless Spiral of Applications

I bet you know what the Fibonacci Sequence is. You know, the sequence where each successive term is the sum of the two previous terms. The first couple of terms look like this: {1, 1, 2, 3, 5, 8, 13, 21, 34, …}. But did you know that it’s actually frequently applied in the real world?

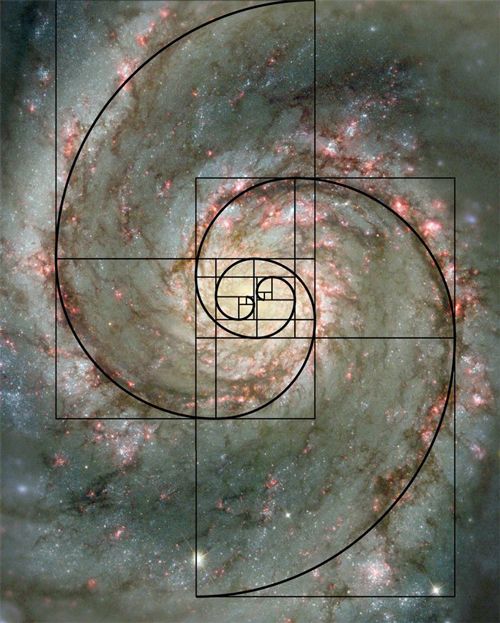
You might think: How could an arbitrary sequence like this be of any importance at all? Actually, there are many examples of it playing a key role across different fields. For example, the spirals of a sunflower and the arrangement of leaves around a stem are packed at maximum efficiency by following the Fibonacci sequence. This allows for the plant to be exposed to as much sunlight and rain as possible, both of which are key to its growth.

The sequence doesn’t only apply to nature; it’s also applied in human-made objects for aesthetics. By dividing each term by its previous term, the result gets closer and closer to 1.618. Using this ratio, many artists and architects created works that are pleasing to the eye, including the Parthenon in Athens and the Apple logo.

There are many other applications of the Fibonacci sequence. If you’re interested, check out Patterns, Mathematics, And Real-World Applications Of Fibonacci Numbers by SmoresScience. 

Hope you found this month’s Monthly Math Morsel interesting! See you next month!